Divide by Fractions

by

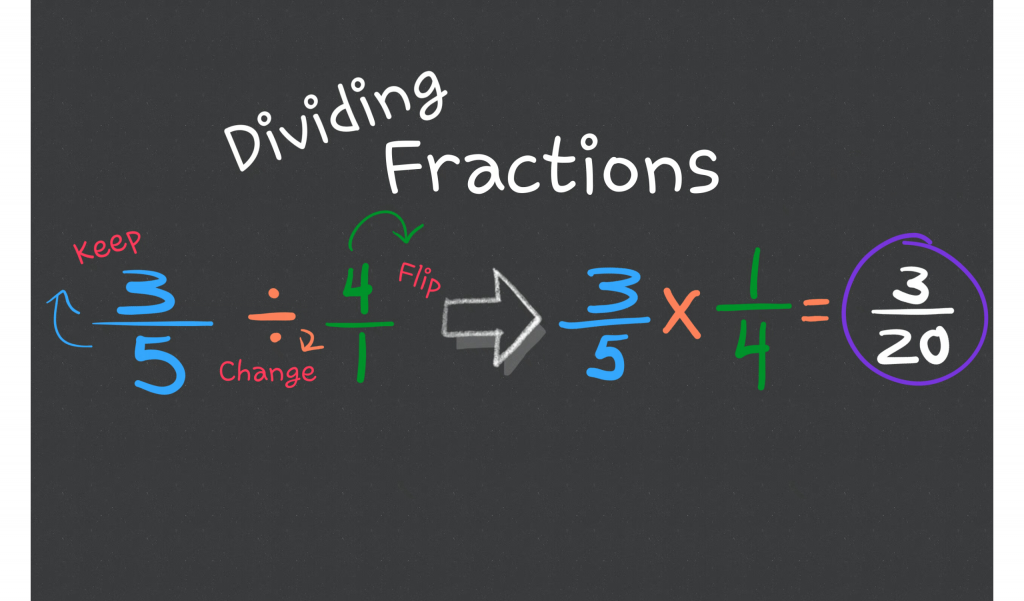
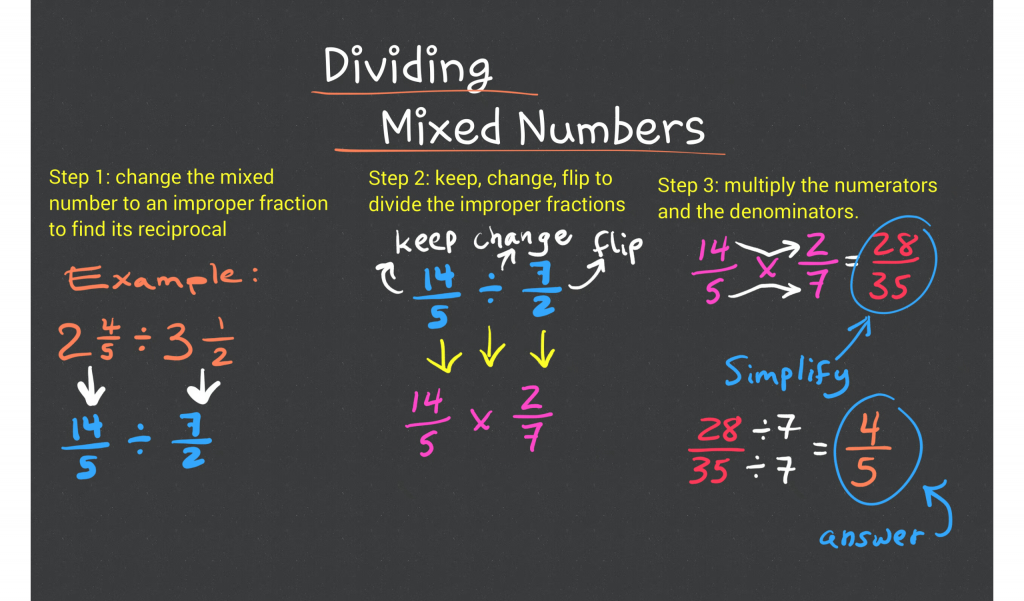
How to Divide by Fractions:

Multiplying by the reciprocal

Example with a fraction being divided by a fraction

problem:   \frac{5}{8}\div \frac{2}{4}

Step 1: turn the fraction you are dividing by upside-down (this gives you its’ reciprocal) and flip the division sign into a multiplication sign.
\frac{5}{8}\times\frac{4}{2}

Step 2: Multiply across

5×4=20 ; 8×2=16     So,  \frac{5}{8}\times\frac{4}{2}=\frac{20}{16}

Step 3: Simplify

\frac{20}{16}\div\frac{4}{4}=\frac{5}{4}
If you end up with an improper fraction, make sure to keep simplifying

\frac{5}{4}=1\frac{1}{4}

 

Example with a whole number being divided by a fraction

Problem:   4\div\frac{3}{4}

*Before you begin, understand that every whole number has an “invisible” denominator of “1”
For example, 8=\frac{8}{1}, 6=\frac{6}{1}, etc.

Multiply using the reciprocal

4\div\frac{3}{4}=\frac{4}{1}\times\frac{4}{3}=\frac{16}{3}

Doing so, we get an answer of  \frac{16}{3}

The fraction \frac{16}{3}is an improper fraction (the numerator is greater than the denominator).

While there is nothing incorrect about this, an improper fraction is typically
simplified further into a mixed number.

The whole number part of the mixed number is found by dividing the 16 by the 3.
In this case we get 5

The fractional part of the mixed number is found by using the remainder of the division,
which in this case is 1 (16 divided by 3 is 5 remainder 1).

The final answer is: 5\frac{1}{3}

 

Example with a mixed number being divided by a fraction

problem:   2\frac{5}{9}\div\frac{3}{5}

To start, we first need to convert 2\frac{5}{9} to an improper fraction.

To convert 2\frac{5}{9} to an improper fraction:
multiply the 9 (the denominator), and the 2 (the whole number). To this product, add the 5 (the numerator)
giving 23, to form the new numerator, and use the 9 as the new denominator.

So, 2\frac{5}{9} as an improper fraction is \frac{23}{9}

The problem here is to divide  and

This problem can be solved by multiplying \frac{23}{9}  by the reciprocal of \frac{3}{5}

To find the reciprocal of \frac{3}{5}
Simply exchange the numerator and denominator, or just “flip” the fraction
upside-down.

The reciprocal of \frac{3}{5} is \frac{5}{3}

The problem here is to multiply \frac{23}{9} and \frac{5}{3}
This problem can be solved by multiplying together the two numerators (the 23 and 5),
giving 115, which will be the numerator in our answer.

Also, we’ll multiply together the two denominators (the 9 and 3),
giving 27, which will be the denominator in our answer.

Doing so, we get an answer of \frac{115}{27}

The fraction \frac{115}{27} is an improper fraction (the numerator is greater than the denominator).
While there is nothing incorrect about this, an improper fraction is typically
simplified further into a mixed number.

The whole number part of the mixed number is found by dividing the 115 by the 27.
In this case we get

The fractional part of the mixed number is found by using the remainder of the division,
which in this case is 7 (115 divided by 27 is 4 remainder 7).

The final answer is: 4\frac{7}{27}

dividing fractions

dividing mixed numbers

Leave a Comment